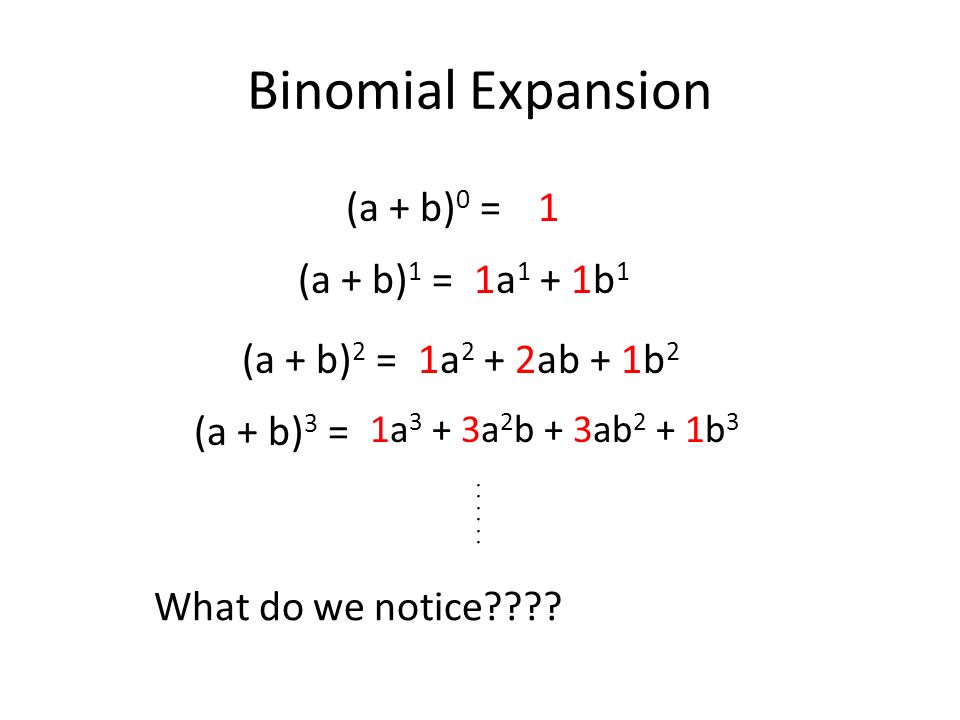
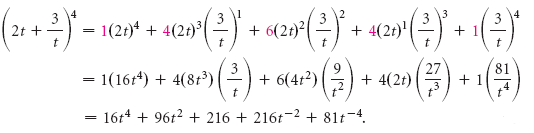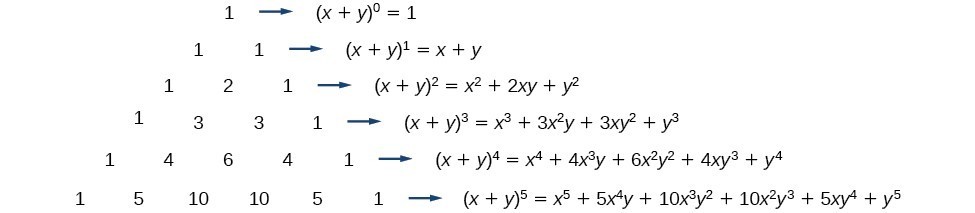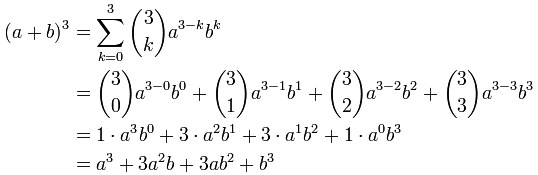A B 3 Binomial Expansion

Hspace 1 5em a 3 3 a 2 b 3a b 2 b 3 of course this is a really tedious way for a very large index power exponent number. In elementary algebra the binomial theorem or binomial expansion describes the algebraic expansion of powers of a binomial according to the theorem it is possible to expand the polynomial x y n into a sum involving terms of the form ax b y c where the exponents b and c are nonnegative integers with b c n and the coefficient a of each term is a specific positive integer depending. A 2 2ab b 2 a b a 3 3a 2 b 3ab 2 b 3.

model lucrare de licenta asistent medical generalist mirela boureanu vaida alexandru vaida mo dao zu shi season 2 mo dao zu shi season 2 episode 1 watch online model de cerere de motivare a absentelor modele de unghii cu gel 2020 albastre modele de garduri din beton si fier forjat model acord privind prelucrarea datelor cu caracter personal scoala

The Binomial Theorem Binomial Expansions Using Pascal S Triangle Subsets

A B 0 1 A B 1 A B 2 A B 3 1a 1 1b 1 1a 2 2ab 1b 2 1a 3 3a 2 B 3ab 2 1b 3 Binomial Expansion What Do We Notice Ppt Download

The Binomial Theorem 1

Binomial Expansion Additional Maths

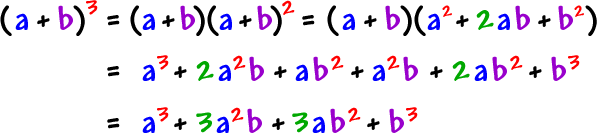
What Is The Formula Of Math A B 3 Math Quora

A b a b a 2 2ab b 2 now take that result and multiply by a b again.

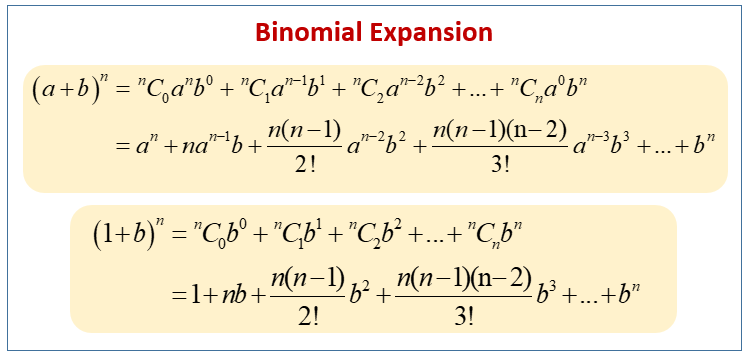
A b 3 binomial expansion. The binomial theorem is used to describe the expansion in algebra for the powers of a binomial. Let us multiply a b by itself using polynomial multiplication. Find the coefficient of a 3 b 2 c 4 d in the expansion of a b c d 10. Instead of doing it manually we could use a formula called the binomial theorem which is shown below.

Thus the formula for the expansion of a binomial defined by binomial theorem is given as. Free math problem solver answers your algebra geometry trigonometry calculus and statistics homework questions with step by step explanations just like a math tutor. The binomial theorem defines the binomial expansion of a given term. In general you can skip the multiplication sign so 5x is equivalent to 5 x.

Find the ratio of the 5 th term from the beginning to the 5 th term from the end in the binomial expansion of 2 1 3 1 2 3 1 3 10. In general you can skip parentheses but be very careful. A b n sum k 0 n begin pmatrix n k. A b a b is a binomial the two terms are a and b.

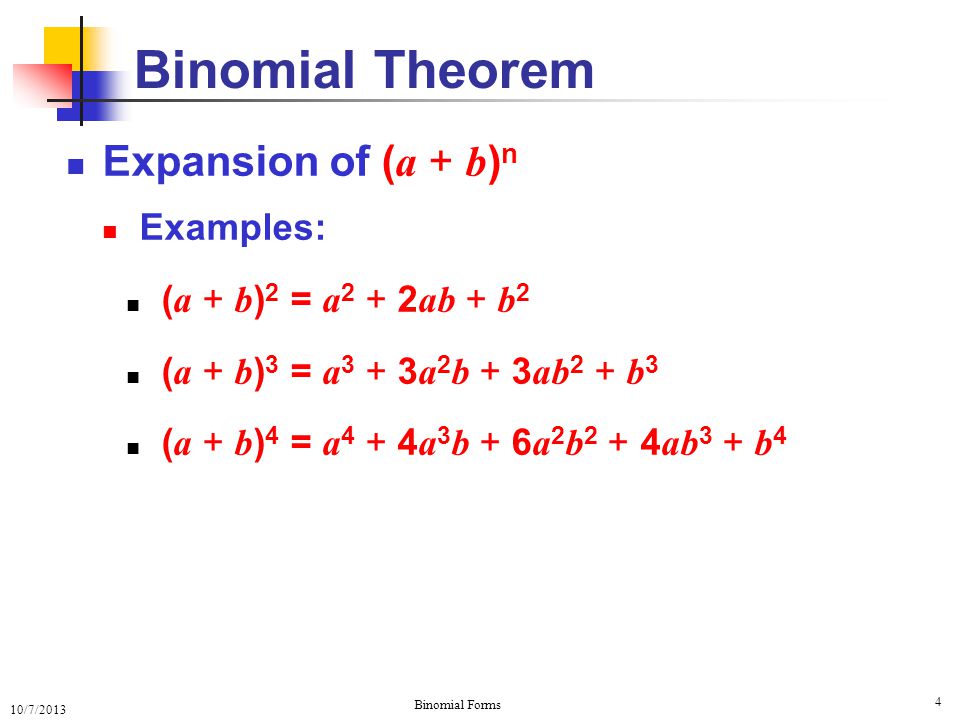
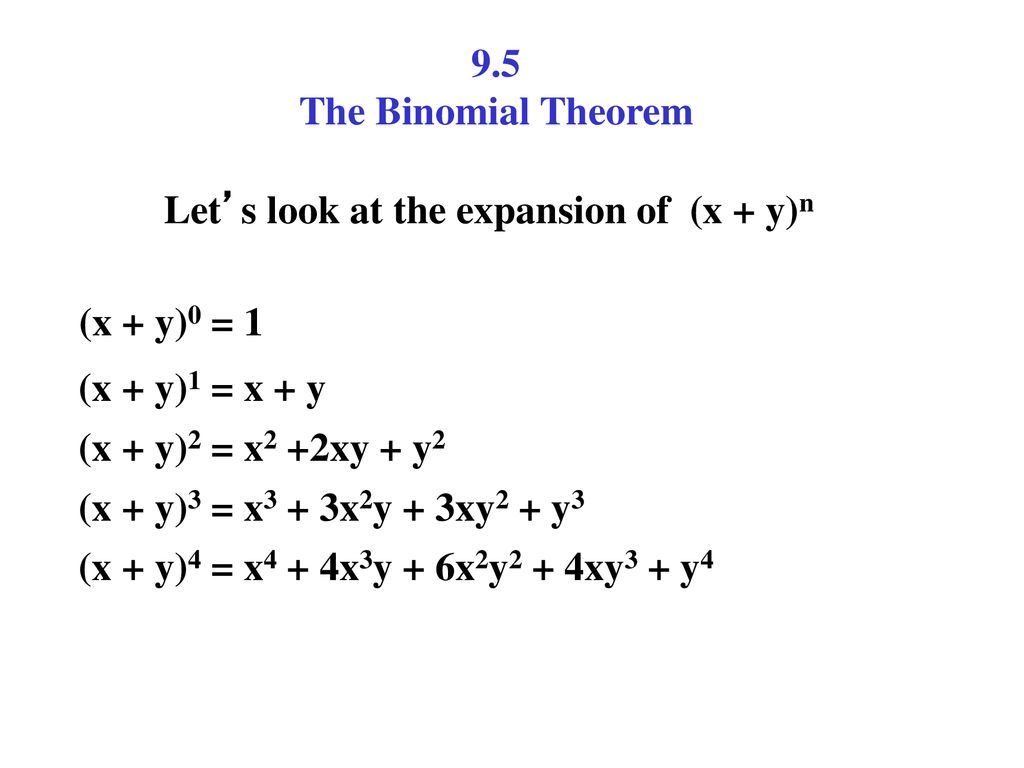
A binomial theorem is a mathematical theorem which gives the expansion of a binomial when it is raised to the positive integral power. A 3 3a 2 b 3ab 2 b 3 a b a 4 4a 3 b 6a 2 b 2 4ab 3 b 4. E 3x is e 3x and e 3x is e 3x. According to this theorem it is possible to expand the polynomial x y n into a series of the sum involving terms of the form a x b y c.

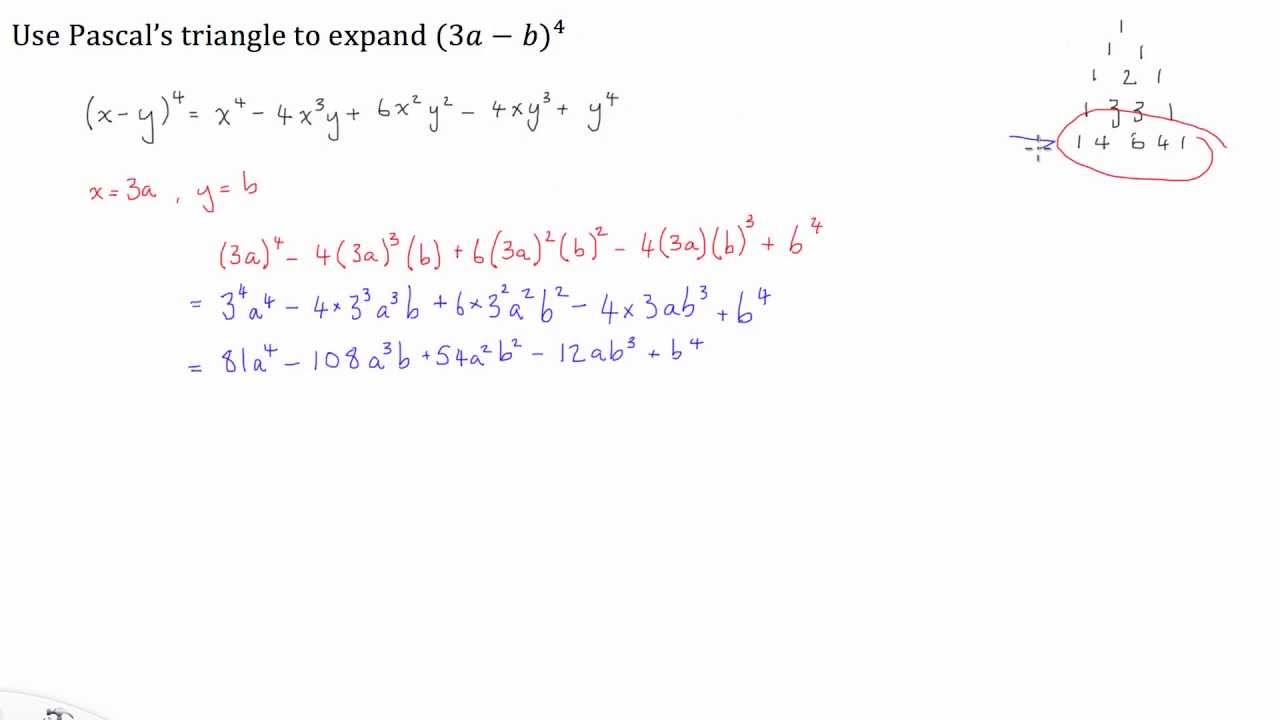
The calculator will find the binomial expansion of the given expression with steps shown.